Paul Krugman, Nick Rowe and an Endogenous Money Model
By Steve Keen
Paul Krugman posted on a familiar topic yesterday—the failure of most inflation hawks to admit that they were wrong—and included praise for one such hawk who has indeed changed his mind and said so:
There’s an interesting contrast with one of the real intellectual heroes here, Narayana Kocherlakota of the Minneapolis Fed, who has actually reconsidered his views in the light of overwhelming evidence. In our political culture, this kind of switch is all too often made into an occasion for gotchas: you used to say that, now you say this. But learning from experience is a good thing, not a sign of weakness. (“A Tale of Two Fed Presidents”)
If this was all there was to the article, I would have finished the (as usual) enjoyable read, and moved on to the next link from Twitter. But then there was this statement:
Look, some of us came into the crisis with a more or less fully formed intellectual framework — extended IS-LM (with endogenous money) — and substantial empirical evidence from Japan…
Huh? “extended IS-LM (with endogenous money)”??? Paul has of course exposited on and promoted IS-LM many times in the past. But “with endogenous money”? Normally this is something he has derided. In the past, his perspective has been “IS-LM with Loanable Funds”, not “with Endogenous Money”.
Now I could be reading too much into this phrase. As Nick Rowe said in the very intelligent and civil discussion on his excellent post “What Steve Keen is maybe trying to say”, the phrase can mean different things to different people:
I don’t find the “exogenous vs endogenous money” distinction helpful in this context, simply because different people seem to mean many different things by that. (Nick Rowe)
Paul could mean something quite different to what I mean by “endogenous money” too, and I could be reading far too much into this single phrase (heck, it could even be a typo!). But if he is shifting his position in the “money and banks don’t matter” and “money and banks are crucial” debate, even a little, that’s something to be applauded in precisely the same manner in which he praised Kocherlakota for moving on inflation. And if not—if he meant something entirely different to the interpretation I put on that statement—well then doubtless we’ll find out. We’ll surely get a clarification in due course.
Whatever that clarification turns out to be, this unexpected phrase has motivated me to publish, ahead of schedule, a model comparing Loanable Funds to Endogenous Money that I promised to provide in the discussion on Nick’s blog:
If the lender is a non-bank, then the repayment of a debt lets the lender spend because both debt and loan are on the liability side of the banking system’s ledger; but if the lender is a bank, then the repayment of the loan takes money out of circulation (I prefer that expression to “destroys money”) because the debt is on the asset side of the ledger. That’s the essential difference between Loanable Funds & Endogenous Money, which I’m trying to illustrate in a pair of very simple models that I’ll post on my blog shortly—and link to Nick’s discussion here. (A comment by me on Nick Rowe’s post)
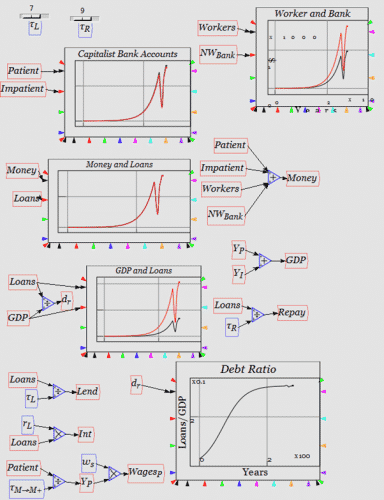
I have since developed that pair of models; there’s much more analysis needed before I’m willing to publish an academic paper on the topic, but here’s what I think is the simplest possible model contrasting Loanable Funds—in which banks, money and (except during a liquidity trap) private debt don’t matter to macroeconomics—and Endogenous Money—in which banks, debt and money are crucial to macroeconomics at all times.
A monetary model of Loanable Funds
My starting point is Krugman’s description of the essence of lending as being a transfer between Patient and Impatient agents:
Think of it this way: when debt is rising, it’s not the economy as a whole borrowing more money. It is, rather, a case of less patient people—people who for whatever reason want to spend sooner rather than later—borrowing from more patient people. (Paul Krugman, 2012, pp. 146–47. Emphasis added)
In the New Keynesian model of a liquidity trap that he and Eggertsson developed (Gauti B. Eggertsson and Paul Krugman, 2012), lending was for the purposes of consumption, and while there was debt, there were neither banks nor money:
We assume initially that borrowing and lending take the form of risk-free bonds denominated in the consumption good. (Gauti B. Eggertsson and Paul Krugman, 2012, p. 1474)
My model of Loanable Funds embeds this vision of lending as being a transfer from Patient to Impatient agents in a monetary model of the economy—one in which all transactions involve the transfer of money in bank accounts—and one in which “Patient agents” and “Impatient agents” are both capitalists, who need money to hire workers and also buy inputs from each other. Though the banking sector exists in this model it is entirely passive: it is just where “Patient agents” deposit their cash, and lending is seen as a transfer from the deposit accounts of the Patient agents to the deposit accounts of the Impatient agents.
I also treat lending as being primarily for production rather than consumption. Both Patient and Impatient agents are capitalists who need money to hire workers and buy inputs for production (as well as for consumption) from each other. The basic financial operations are:
- An initial deposit of 90 by the Patient agents and loan to the Impatient agents of 10, both of which are stored in bank accounts;
- Lending by the Patient Agents to the Impatient Agents;
- Payment of interest;
- Repayment of the debt;
- Hiring of workers by both groups of agents;
- Consumption by all agents from both Patient and Impatient.
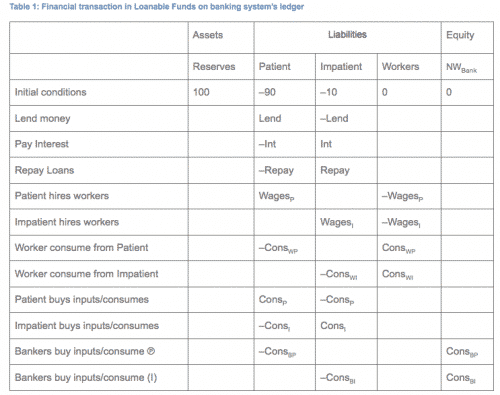
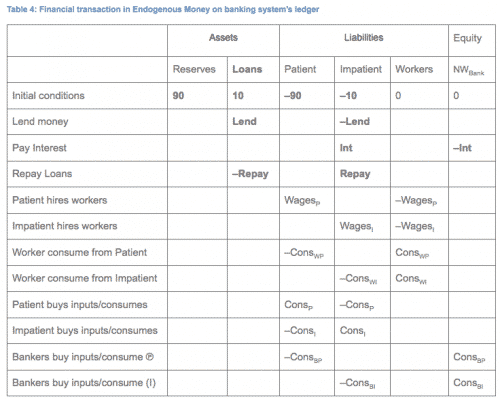
The operations are shown below in Table 1, following the conventions in the Minsky program that assets are shown as positives, liabilities and equity are shown as negatives, the source of any flow is a positive and its destination is a negative: these conventions ensure that all rows have to sum to zero to be correct in accounting terms (the program also supports the accounting approach of using DR and CR).
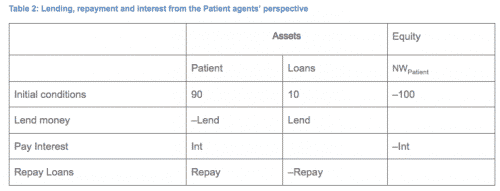
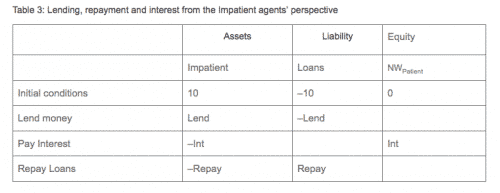
Loans themselves don’t turn up on the banking sector’s ledger because they are transfers between the Patient and Impatient agents. Instead loans are assets of the Patient agents and a liabilities of the Impatient agents. Table 2 shows Loans from the Patient agents’ perspective, while Table 3 shows the same operations from the Impatient agents’ perspective.
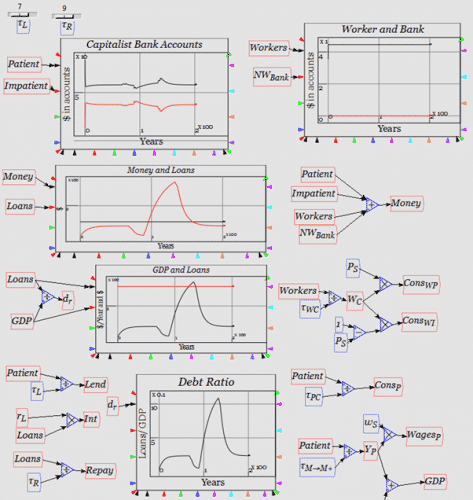
The Minsky program (click here for the latest beta build) is primarily designed for numerical simulation—and I’ll get to that shortly—but it also generates the dynamic equations in the model, and they are instructive enough for those who don’t suffer the MEGO effect (“My Eyes Glaze Over”) when looking at equations (if you do, skip most of this and just check the simulations below). The equations of motion of the key accounts in this model (click here to download the model) are shown in Equation . The first four equations describe the dynamics of money in this model; the last equation describes the dynamics of debt.
The key points from these equations are:
- The total amount of money in the system is the sum of the four accounts Impatient, Patient, Workers and NWBank (for “Net Worth of the Banking sector”, which is zero in this model), and this doesn’t change: the sum of the first 4 equations is zero:
- The total amount of money in the firm sector is the sum of the first two accounts—Patient and Impatient—and the annual turnover of this amount is the annual GDP of this model. It is unaffected by lending, repayment and debt service, so GDP is also unaffected by lending, repayment and debt service:
- Finally, the dynamics of debt in this model are
This structure means that, no matter what behavioral relations are used to model lending, repayment, consumption, etc., changes in the level of debt have no impact on the macroeconomy. This is confirmed by the relations I used to simulate this model, which used simple time constants to specify all flows. In Figure 1 I ran the model with a time constant of 7 years for lending and 9 years for repayment until the debt to GDP ratio stabilized at 0.24 (which took about 60 years), and then altered time constants—firstly simulating a slump in lending, then a boom, and finally a return to the initial rates. The level of debt and the debt to GDP ratio varied dramatically, but GDP sailed on undisturbed. So if Loanable Funds accurately characterized actual lending, banks, money and (except during a liquidity trap) debt would indeed by irrelevant to macreoeconomics.
Figure 1: Simulation of Loanable Funds
Endogenous Money proponents, on the other hand, insist that most lending is not between non-banks, but from banks to non-banks, and that this makes all the difference in the world. That is easily illustrated by making just 3 simple changes to this model:
- Lending is shown as being a flow from Banks to Impatient Agents;
- Interest payments go not from Impatient to Patient but from Patient to the NWBank; and
- When the model is simulated, lending is related to the current level of lending rather than to the amount of money in the Patient Agents’ accounts.
So what difference did these simple structural changes make? A lot.
A monetary model of Endogenous Money
The monetary system from the banking sector’s point of view is shown in Table 4: Loans are now an asset of the banking sector, while lending, repayment and debt service are all relations between the Impatient agents and the banking sector. The differences of this model with Loanable Funds are highlighted in bold in the Table (click here to download the model).
The equations of motion of this system are:
There are three significant ways in which this model differs from Loanable Funds:
- The total amount of money in the system is, as before, the sum of the four accounts Impatient, Patient, Workers and NWBank (for “Net Worth of the Banking sector”, which is not zero in this model), and this now is altered by the change in debt:
- The total amount of money in the firm sector is the sum of the first two accounts—Patient and Impatient—and the annual turnover of this amount is the annual GDP of this model. It is also altered by lending, repayment and debt service, and therefore so is GDP:
- Finally, the dynamics of debt in this model are the same as in Loanable Funds, but now this is also identical to the dynamics of the money supply:
I simulated the model for 250 years with constant parameters (it took that long for the debt to GDP ratio to stabilize at 0.32), and then repeated the experiment of a slump in lending followed by a boom and then a return to normality. The results in Figure 2 shows how different an Endogenous Money view of the world is to Loanable Funds.
Firstly, in Endogenous money, the growth of debt is not macroeconomically neuteal but causes GDP to grow: rather than the change in debt being irrelevant to the macroeconomy as in Loanable Funds, in Endogenous Money it alters the level of demand. Secondly, alterations in the rate of change of debt had drastic effects on the economy: a decline in lending caused a slump and an increase in lending caused a boom.
Figure 2: Simulation of Endogenous Money
IS-LM and Endogenous Money?
If—and it’s a big if—this phrase signifies a shift in how Krugman models IS-LM, then it will surely mean something very different to what I’ve shown above. For starters, it’s likely to be an equilibrium model, when as Nick Rowe rightly concluded, my story is a disequilibrium one (something that Hicks argued long ago can’t be done with IS-LM—see (John Hicks, 1981)):
We are talking about a Hayekian process in which individuals’ plans and expectations are mutually inconsistent in aggregate. We are talking about a disequilibrium process in which people’s plans and expectations get revised in the light of the surprises that occur because of that mutual inconsistency. (Nick Rowe)
Of course, it could signify no more than a rebadging of Krugman’s established approach—or even a typo. We’ll have to await an elaboration. But I do hope that it does signify a further thawing in the relations between orthodox economists and those from the non-orthodox end of the spectrum after Nick Lowe’s recent contribution.
Nick’s post—a reminder
As I acknowledged in “An outbreak of communication”, Nick’s post accurately stated my arguments on the creation of aggregate (or effective) demand via the creation of money by loans from the banking system to the public:
So with that very big caveat understood, here’s what I think Steve Keen is maybe trying to say:
Aggregate planned nominal expenditure equals aggregate expected nominal income plus amount of new money created by the banking system minus increase in the stock of money demanded. (All four terms in that equation have the units dollars per month, and all are referring to the same month, or whatever.)
And let’s assume that people actually realise their planned expenditures, which is a reasonable assumption for an economy where goods and productive resources are in excess supply, so that aggregate planned nominal expenditure equals aggregate actual nominal expenditure. And let’s recognise that aggregate actual nominal expenditure is the same as actual nominal income, by accounting identity. So the original equation now becomes:
Aggregate actual nominal income equals aggregate expected nominal income plus amount of new money created by the banking system minus increase in the stock of money demanded.
Nothing in the above violates any national income accounting identity. (Nick Rowe)
This is, from my perspective, the essence of the significance of Endogenous Money. If this wasn’t true—if the creation of new money by the banking system didn’t somehow impact on actual income and demand—then by Occam’s Razor, there would be no macroeconomic significance to Endogenous Money, and we’d be better off ignoring the banking sector in macroeconomics, as the model of Loanable Funds does. Nick provided an excellent verbal statement of this—and a logical argument behind it which I think any economist should be able to follow, regardless of his/her school of thought:
Start with aggregate planned and actual and expected income and expenditure all equal. Now suppose that something changes, and every individual plans to borrow an extra $100 from the banking system and spend that extra $100 during the coming month. He does not plan to hold that extra $100 in his chequing account at the end of the month (the quantity of money demanded is unchanged, in other words). And suppose that the banking system lends an extra $100 to every individual and does this by creating $100 more money. The individuals are borrowing $100 because they plan to spend $100 more than they expect to earn during the coming month.
Now if the average individual knew that every other individual was also planning to borrow and spend an extra $100, and could put two and two together and figure out that this would mean his own income would rise by $100, he would immediately revise his plans on how much to borrow and spend. Under full information and fully rational expectations we couldn’t have aggregate planned expenditure different from aggregate expected income for the same coming month.
But maybe the average individual does not know that every other individual is doing the same thing. Or maybe he does know this, but thinks their extra expenditure will increase someone else’s income and not his. Aggregate expected income, which is what we are talking about here, is not the same as expected aggregate income. The first aggregates across individuals’ expectations of their own incomes; the second is (someone’s) expectation of aggregate income. It would be perfectly possible to build a model in which individuals face a Lucasian signal-processing problem and cannot distinguish aggregate/nominal from individual-specific/real shocks.
So at the end of the month the average individual is surprised to discover that his income was $100 more than he expected it to be, and that he has $100 more in his chequing account than he expected to have and planned to have. This means the actual quantity of money is $100 greater than the quantity of money demanded. And next month he will revise his plans and expectations because of this surprise. How he revises his plans and expectations will depend on whether he thinks this is a temporary or a permanent shock, which has its own signal-processing problem. And these revised plans may create more surprises the following month. (Nick Rowe)
Eggertsson, Gauti B. and Paul Krugman. 2012. “Debt, Deleveraging, and the Liquidity Trap: A Fisher-Minsky-Koo Approach.” Quarterly Journal of Economics, 127, 1469–513.
Hicks, John. 1981. “Is-Lm: An Explanation.” Journal of Post Keynesian Economics, 3(2), 139–54.
Krugman, Paul. 2012. End This Depression Now! New York: W.W. Norton.
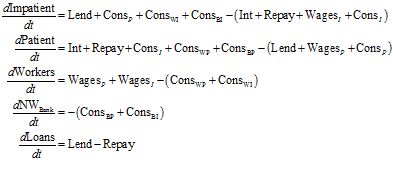

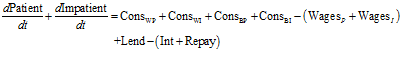
Comments are closed.